Plugging the Right Numbers Into Energy Calculations
(Thanks to Robert Lindquist, whose book* straightened me out on some of these matters.)
Chemistry Department University of Southern Maine rhodes@usm.maine.edu
Consider the reaction 2 NADH + 2H3O+ + O2 --> 2 NAD+ +4 H2O
occurring in aqueous solution at pH 7.6, [NAD+] = 20.0 mM, [NADH] = 10.0 mM, and oxygen at a partial pressure of 100.0 torr.
The free-energy change for this oxidation of NADH by oxygen is
DG = DG° + R•T•ln
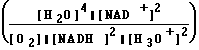
What numbers should you plug in for the concentration terms [ ? ]?
These terms are properly activities, not concentrations. To convert each concentration into activity, divide it by its standard concentration (at standard conditionsT = 298.16 °K, p = 101.3 kPa). This eliminates all units ? within the logarithm term(, because each quantity is divided by a standard concentration in the same units.) Here are the details for each type of reactant and product:
Solutes
For dilute (ideal) solutions, the standard state of the solute IS its molar (not millimolar) concentration. So in this calculation, plug in [NAD+] = 0.020 M, and [NADH] = 0.010 M.
Gases
The standard state for a gas is a pressure of 1 atm or 760 torr. So in this calculation, plug in [O2]=100 torr/760 torr = 0.132 atm in blood plasma disoluted [O2]=5•10-6 M.
Hydrogen Ion
The biochemical standard state for hydrogen ion is pH 7, or 10-7 M. If the pH is 7.36,
[H+]=10-(7.36). So in this calculation, plug in [H+]=10-(7.36).
NOTE: The prime ['] on DG0' implies that we are using biochemical standard states rather than conventional thermodynamic standard states. (In thermodynamics, the standard state for the hydrogen ion is pH = 0 ([H+]=1.00 M).)
Water
The standard state for water is pure water, whose concentration is 55.5 M. In dilute aqueous solutions, the concentration of water is very close to 55.5 M. So in this calculation, plug in
[H2O] = 55.5 M. (NOTE: In a cell, the total solute concentration is high, so the concentration of water is certainly lower than 55.5 M. Nevertheless, biochemists commonly use 55.5 M as the activity of water.)
Calculation of the RT ln() Term:
DG = DG° + 8.314 J/mol/K•310.16 °K•ln
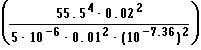
DG = DG° + 15.3 kJ/mol.
(For an example of DG° calculation, see Summary of Energy Calculations.) Summary