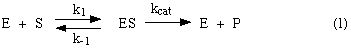Deriving the Michaelis-Menten Equation Gale Rhodes
Chemistry Department University of Southern Maine rhodes@usm.maine.edu
   Memorize this derivation as soon as your encounter it in your text, and you will be able to read the remainder of the chapter with far greater understanding. For other suggestions on how to make your study of biochemistry easier, see Learning Strategies.
A simple model of enzyme action: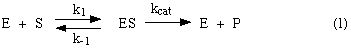
We would like to know how to recognize an enzyme that behaves according to this model. One way is to look at the enzyme's kinetic behavior -- at how substrate concentration affects its rate. So we want to know what rate law such an enzyme would obey. If a newly discovered enzyme obeys that rate law, then we can assume that it acts according to this model. Let's derive a rate law from this model.
For this model, let V₀ be the initial velocity of the reaction. Then V₀ = k_cat [ES]. (2)
   The maximum velocity V_maxoccurs when the enzyme is saturated -- that is, when

all enzyme molecules are tied up with S, or   [ES] = [E]_total .
So V_max = k_cat [E]_total. (3)
   We want to express V₀ in terms of measurable quantities, like [S] and [E]_total , so we can see how to test the mechanism by experiments in kinetics. So we must replace [ES] in (2) with measurables.
   During the initial phase of the reaction, as long as the reaction velocity remains constant, the reaction is in a steady state, with ES being formed and consumed at the same rate. During this phase, the rate of formation of [ES] equals its rate of consumption. According to model (1), Rate of formation of [ES] = k₁[E][S].
Rate of consumption of [ES] = k_-1[ES] + k_cat [ES].
So in the steady state, k_-1[ES] + k_cat [ES] = k₁[E][S]. (4)
   Remember that we are trying to solve for [ES] in terms of measurables, so that we can replace it in (2). First, collect the kinetic constants in (4): (k_-1+k_cat)[ES]=k₁[E][S],
and (k_-1 + k_cat)/k₁ = [E][S]/[ES]. (5)
   To simplify (5), first group the kinetic constants by defining them as K_m : K_m=(k_-1+k_cat)/k₁(6) and then express [E] in terms of [ES] and [E]_total: [E]=[E]_total-[ES] (7)  Substitute (6) and (7) into (5): Km=([E]_total-[ES]) [S]/[ES] (8)
Solve (8) for [ES]: First multiply both sides by [ES]: [ES] K_m=[E]_total[S]-[ES][S]Then collect terms containing [ES] on the right: [ES]K_m+[ES][S]=[E]_total[S]
Factor [ES] from the left-hand terms: [ES](K_m+[S])=[E]_total[S] and finally, divide both sides by (K_m + [S]): [ES]=[E]_total[S]/(K_m+[S]) (9)
Substitute (9) into (2): V₀ = k_cat [E]_total [S]/(K_m + [S]) (10)
Recalling (3), substitute V_max into (10) for k_cat [E]_total: V₀=V_max [S]/(K_m+[S]) (11)
   This equation expresses the initial rate of reaction in terms of a measurable quantity, the initial substrate concentration. The two kinetic parameters, V_maxand K_m , will be different for every enzyme-substrate pair.
   Equation (11), the Michaelis-Menten equation, describes the kinetic behavior of an enzyme that acts according to the simple model (1). Equation (11) is of the form
y = ax/(b + x) (does this look familiar?)
This is the equation of a rectangular hyperbola, just like the saturation equation for the binding of dioxygen to myoglobin.
   Equation (11) means that, for an enzyme acting according to the simple model (1), a plot of V₀ versus [S] will be a rectangular hyperbola. When enzymes exhibit this kinetic behavior, unless we find other evidence to the contrary, we assume that they act according to model (1), and call them Michaelis-Menten enzymes.
QUIZ for USM Students
Quiz at first class on enzyme kinetics: Derive equation (11) from model (1).
Ten minutes.
Back to Goodies List  Back to Biochemistry Resources  HOME